2 Stressor-Response Functions

Stressor-response functions describe the relationship between a specific stressor (such as habitat loss, temperature, or a specific pollutant) and the response of a target species, where responses can include abundance, growth rate, reproduction, or mortality ((Rosenfeld et al., 2024); (Jarvis et al., 2023)). Stressor-response functions are used to predict how a population (or study system) will respond to changes in the environment and to help identify thresholds or “critical levels” at which a stressor becomes harmful. Stressor-response functions are often used to inform environmental policy and management decisions, for example, by identifying risk levels of pollution or temperature change for a particular species or ecosystem¹. Stressor-response functions are generally developed through primary research (i.e., mechanistic, empirical, experimental etc.) and expert opinion ((Pirotta et al., 2022); (Jarvis et al., 2023)). Note, however, that stressor-response functions are often continuous empirical or mechanistic relationships and identification of specific thresholds as harmful or benign will often be a subjective user-defined activity for stressors and responses that are without direct regulatory guidance (e.g., habitat area, population size).

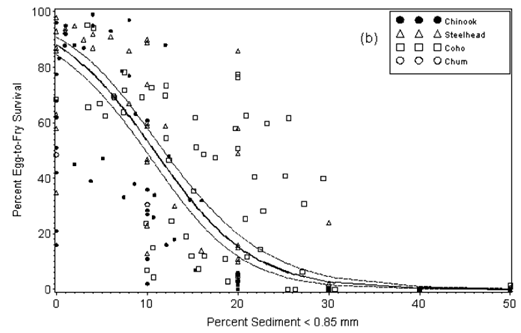
Figure 2.1: An example of a stressor-response function for Pacific salmon from Jensen et al. (2009) shows the relationship between a stressor (percent fine sediment) on the x-axis and the biological response (percent egg-to-fry survivorship) on the y-axis.

There are many types of stressor-response functions, including linear, threshold, and non-linear ((Rosenfeld, 2017); (Larned & Schallenberg, 2019)). Linear functions describe a simple, linear relationship between the stressor and the response, with the response increasing or decreasing at a constant rate as the stressor increases. Threshold functions describe a breakpoint at which a stressor becomes harmful, beyond which the response increases rapidly. Non-linear functions describe more complex relationships, with the response changing at different rates as the stressor increases. The example provided in Figure 2.1 shows a customized non-linear stressor-response function fit to empirical data (reference points). Stressors do not always act independently ((Schäfer & Piggott, 2018); (Jarvis et al., 2023)), and it is also possible to include interactions among variables in stressor-response functions, such as the risk of exposure to a harmful pathogen being temperature dependent.

For a more in-depth discussion on the foundations of stressor-response functions, refer to the following resources:

(Rosenfeld et al., 2024); (Jarvis et al., 2023): Conceptual overviews of stressor-response functions as a generalizable model for context dependence. This paper provides a valuable overview to conceptualize stressors as a mechanism to characterize the state of a system and ecological process. (Jarvis et al., 2023) also outline common forms of stressor-response functions and key considerations for the creation of a stressor-response function from empirical data.
(Piet et al., 2021): A roadmap towards quantitative cumulative impact assessments: Every step of the way. Provides an important roadmap for working groups to consider linkages between land-use activities, resulting ecosystem pressures, functional linkages in space and time (exposure) and the consideration of endpoints to target study systems.
Incorporating Indigenous Knowledge: In many instances, stressor-response functions may be developed through expert opinion from local communities based on value systems. Where appropriate, working groups may include a customized stressor-response function to represent potential risks and values based on traditional knowledge systems and expert opinion. Refer to (Houde, 2007) and (Alexander et al., 2019) for further discussion. Examples of many other values-based Indigenous-led cumulative effects management programs exist across Canada.

Alexander, S. M., Provencher, J. F., Henri, D. A., Taylor, J. J., Lloren, J. I., Nanayakkara, L., et al. (2019). Bridging indigenous and science-based knowledge in coastal and marine research, monitoring, and management in canada. Environmental Evidence, 8(1), 1–24.

Houde, N. (2007). The six faces of traditional ecological knowledge: Challenges and opportunities for canadian co-management arrangements. Ecology and Society, 12(2).

Jarvis, L., Rosenfeld, J., Gonzalez-Espinosa, P., & Enders, E. (2023). A process framework for integrating stressor-response functions into cumulative effects models. Science of the Total Environment, XXX, 167456.

Jensen, D. W., Steel, E. A., Fullerton, A. H., & Pess, G. R. (2009). Impact of fine sediment on incubation survival of pacific salmon: A meta-analysis of published studies. Reviews in Fisheries Science, 17(3), 348–359. https://doi.org/10.1080/10641260902716954

Larned, S. T., & Schallenberg, M. (2019). Stressor-response relationships and the prospective management of aquatic ecosystems. New Zealand Journal of Marine and Freshwater Research, 53, 489–512.

Piet, G. J., Tamis, J. E., Volwater, J., Vries, P. de, Wal, J. T. van der, & Jongbloed, R. H. (2021). A roadmap towards quantitative cumulative impact assessments: Every step of the way. Science of The Total Environment, 784, 146847.

Pirotta, E., Thomas, L., Costa, D. P., Hall, A. J., Harris, C. M., Harwood, J., et al. (2022). Understanding the combined effects of multiple stressors: A new perspective on a longstanding challenge. Science of the Total Environment, 821, 153322.

Rosenfeld, J. S. (2017). Developing flow–ecology relationships: Implications of nonlinear biological responses for water management. Freshwater Biology, 62, 1305–1324.

Rosenfeld, J. S., Ayllón, D., Grant, J. W., Naman, S. M., Post, J. R., Matte, J.-M., et al. (2024). Determinants of productive capacity for stream salmonids. In J. Lobon-Cervia, P. Budy, & R. Gresswell (Eds.), Advances in the ecology of stream-dwelling salmonids (pp. 1–12). Springer Life Sciences.

Schäfer, R. B., & Piggott, J. J. (2018). Advancing understanding and prediction in multiple stressor research through a mechanistic basis for null models. Global Change Biology, 24, 1817–1826.

https://www2.gov.bc.ca/assets/gov/environment/air-land-water/water/waterquality/water-quality-guidelines/approved-wqgs/wqg_summary_aquaticlife_wildlife_agri.pdf.↩︎

Leave questions and comments below (via your GitHub account)